2.1.1. Elements of a double line

In contrast to mechanical systems there are only a few cases where an electric current is not flowing in a closed loop like for instance the de-charging of a capacitor or a lightning, neutralizing separated charges in the atmosphere. In all cases of practical importance only closed electrical circuits conists a voltage source with the terminating resistor.
In all cases of practical importance only closed electrical circuits exist with two lines, connecting the voltage source with other cicuit elements, for instance a terminating resistor.
A voltage source is introduced into such a closed circuit at one place to produce an electric current. Such a source will drive electrons from one line to the other. At the other side of the double line we find, as a rule, a resistor, a so-called load, imposing a certain resistance to the drifting flow of electrons.

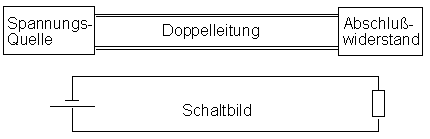
Electric double line and circuit diagram

Similar to a mechanical flow of gas we will make the following assumptions:

• Both parts of an electrical double line are arranged in parallel.
• The distance between them is small compared to their length.

These assumptions lead to following advantages:

All changes perpendicular to the longitudinal dimension can be neglected.

Because of symmetry both lines behave identical with the exception of the direction of flow.

Die in einer solchen linearen Doppelleitung auftretenden A closed theory, developed by Maxwell in the 19. century, exists for a complete determination of all transmission processes on such double lines.
This theory is the base for all simulations used in this tutorial

2.1.2. Transmission velocity on a double line

In a gas or liquid a non-equilibrium state in respect to density or current is equalized by collisions of thermally activated atoms or molecules. Therefore the transmission velocity of any change in current or pressure could not be faster than the thermal velocity of the colliding microscopic particles. For recall: the thermal velocity of air molecules at room temperature and normal pressure is about 450 m/s.
Within an electric system the transmission velocity does not depend on the velocity of the drifting charge carriers. In contrast to neutral molecules charge carriers are surrounded by an electric field. Changes in the density of charge carriers are leading to changes in the electric field and such changes are spread out with the velocity of light v= 300.000 km/s.
On an electric double line pulses of voltage and current are therefore transmitted with a velocity comparable with the velocity of light.
In spite of the fact that the transmission velocity is so many orders of magnitude higher than in mechanical systems, the same principles for transmission processes on a double line are valid. These processes in electric systems will be treated in detail in the following.

2.1.3. Voltage and Current pulses on a double line

Voltage pulse
A double line with a load but without a connected voltage source is ina neutral state and all free electrons are equally distributed. A line with a voltage pulse at a certain place indicates some additional surface electrons at this place on one conductor which are missing on the opposite one.

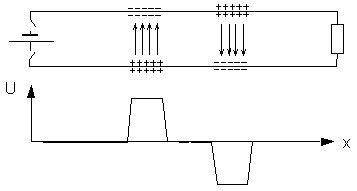
Two voltage pulses of opposite polarity on a double line

Current pulse
The following picture shows a situation which seems difficult to be realized. At a certain moment in time (t = 0) all free electrons within a small zone start drifting in one directions (and in the opposite directions on the other line) with no potential difference between the lines.

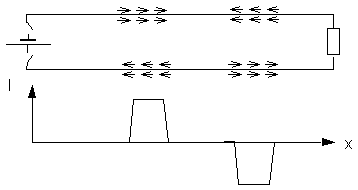
Zones with drifting electrons on a double line

Later it will be seen that such situations can be part of normal processes of reflection and superposition.

2.1.4. Displacement of a voltage pulse on a double line

The following simulation shows a double line with a zone of increased voltage but without any current.
Looking at a microscopic level there are negative charges on the surface of one line, which are missing on the surface of the opposite line. The latter is equivalent with the existance of positive surface charges.

Displacement of a zone with surface charges on a double line
Left: Starting position; Right: Displacement after a few time steps

The negative surface charges repel the other free electrons inside of the wire and each other to both sides, the positive surface charges attract free electrons from both sides. As a result the density of surface charges is reduced in the middle and increased to both sides.
When activating the simulation stepwise it can be seen (like in the mechanical case) that the original voltage zone is split into two wave pulses separating out in both directions.

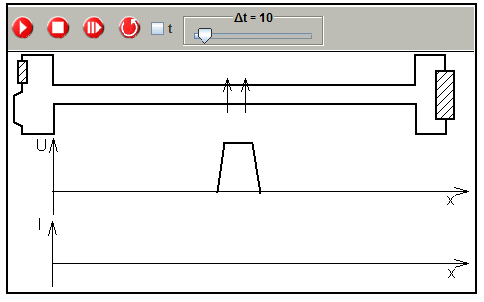
Simulation of a voltage zone on a double line
(prepared simulation: 2-1-4-voltage-puls-transmission.xml)

2.1.5. Displacement of a zone with drifting electrons on a double line

The following figure shows a double line with a narrow zone, where the free electrons start to drift at a certain moment in time (t = t0) with no voltage across the line at that time.

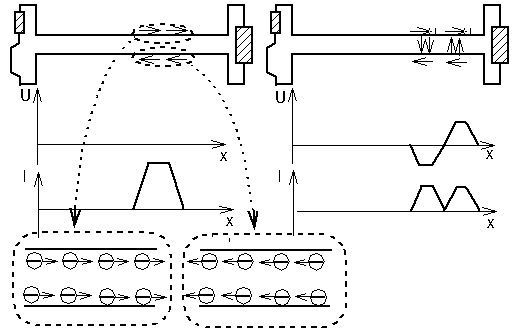
Displacement of a zone with drifting free electrons on a double line
Left: Starting position; Right: Displacement after a few time steps

Due to interaction with the non-drifting electrons in the direction of flow, the concentration of surface charges will increase. Furthermore a drift velocity will result in the direction of the original flow while the originally drifting electrons will slow down.
In the opposite direction the density of electrons will be reduced, leading to the appearance of positve charges on the surface. The free electrons inside of this zone will experience a reduced interaction with the side where the electrons are drifting away and will start drifting in the same direction.
The simulation allows to study this process in detail.

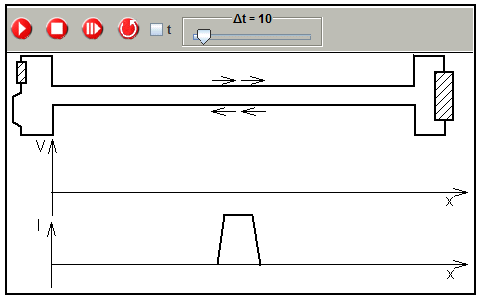
Simulation of a zone with drifting free electrons on a double line
(prepared simulation: 2-1-5-drift-puls-transmission.xml)

It is advised to activate the simulation by using the step button.

2.1.6. Transmission of a voltage/current pulse on a double line

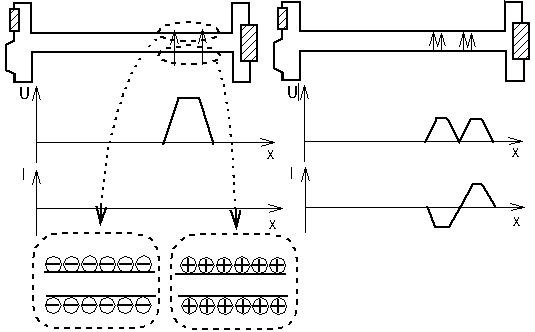
A pulse on a double line consist of a zone with surface charges (with opposite polarity on both lines) as well as drifting electrons (in opposite directionin both lines). Such pulses are the basic elements of every digital data transfer.

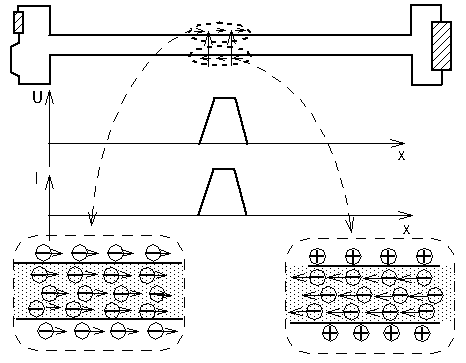
Microscopic view of a pulse on a double line

The same interpretation as in the mechanical case is applicable here for an electric double line.
The two components of a pulse, V and I, spread out to both sides..

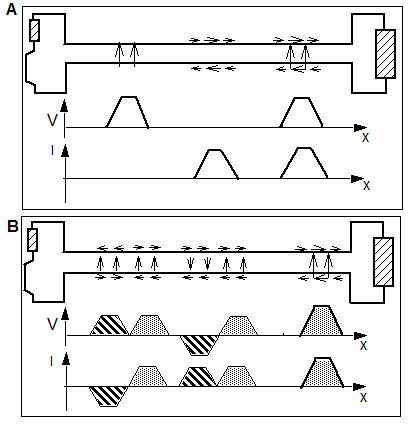
Displacement of a pulse and its components on a double line
A: Starting position; B: Situation after a few time steps

As indicated graphically the displacements of the two components to the left cancel while the displacements to the right add up, resulting in a displacement to the right of the original pulse without change of form.
The simulation allows to study this case in detail.

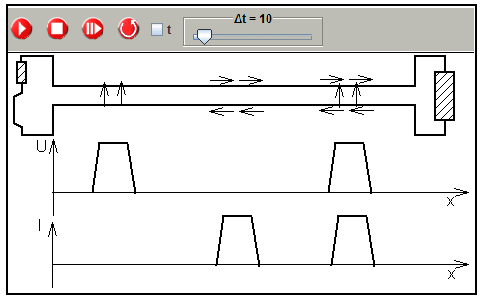
Simulation of the transmission of a pulse
and its components on a double line
(prepared simulation 2-1-6-Puls-with-equal-components.xml)

2.1.7. Transmission of a pulse with an arbitrary ratio of V/I

The following figure shows the displacements of a pulse on a double line together with it´s two components at separate locations. In comparison with the former situations the ratio V/I has been changed.

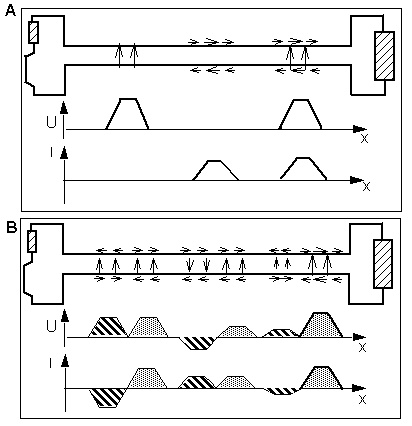
Displacement of a pulse and its components with arbitrary ratio of V/I
A: Starting position; B: Situation after a few time steps

Due to the arbitrary ratio of V/I the displacement of the two components to the left side do not cancel. As a result the original pulse is not transmitted without change of form but is split into two parts, moving in opposite directions.
The simulation allows to study this process in detail.

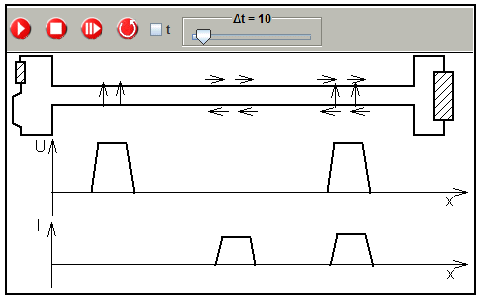
Simulation of a pulse and its components with arbitrary ratio of V/I
(prepared simulation: 2-1-7-Puls-with-different-components.xml)

zurück zur Starseite