back Home
10. Oscillating Motion
10.1. General Properties
Every system which can perform an oscillating motion, has two general properties:
- 1. It has an equilibrium position.
- 2. When the system leaves this position, restoring forces seek to drive the system back to equilibrium.
10.2. Harmonic Oscillator
A special case of an oscillating movement is defined as harmonic if the restoring forces are proportional to the distance from the equilibrium position.
According to Hook's law the following proportion exists between the elongation of a spring s and the resulting force:
This linear relation is in fact the condition stated above for the existence of an harmonic oscillation. A spring is therefore capable of driving an harmonic oscillation.
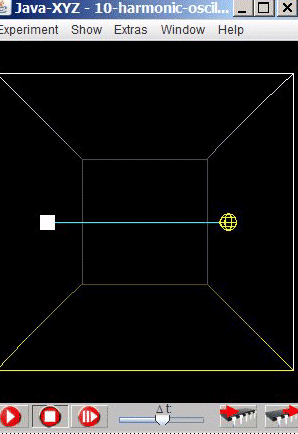
The simulation ,10-harmonic-oscillation" demonstrates such an oscillation, driven by a spring. An important parameter for an oscillation is the time period for one cycle, indicated as T.
Fig 10.1.: Simulation "10-harmonic-oscillation"
|
Fig 10.2.: Spring Editor window
If this procedure is repeated over an existing spring the "Spring Editor" window opens, where spring parameters can be set.
10.3. Harmonic Oscillator and Uniform Circular Motion
The simulation "10-ho-circular-motion" demonstrates that a clear similarity exists between the movement of an harmonic oscillator and a uniform circular movement.
The movement of an harmonic oscillator can be defined as a linear projection of a uniform circular movement.
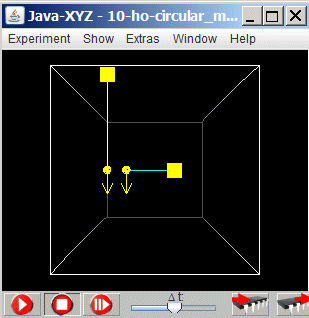
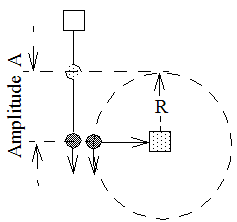
Fig 10.3.: Harmonic oscillation and uniform circular motion
For a uniform circular movement we have:
Period T = 2 π R / v |v| = constant velocity (speed) of the circling object
Since both objects have the same vertical position at any time, the forces, driving in vertical direction, must be equal at any time. Therefore we have for the highest or lowest position:
With R = A we get by substitution and re-arrangement
This proves in accordance with the simulation "10-harmonic-oscillation" the relation: T ∝ √ m/D
This relation also proves that the time T needed for one cycle is independent of the amplitude and therefore also independent of the velocity with which an object starts from the equilibrium position.
10.4. Harmonic Oscillator - Amplitude and Period
Fig 10.4.: Simulation "10-oscillation-amplitude"
The pitch, which is related to the duration of one oscillation, is the same, regardless of the force, with which the glass was struck. A specific glass (with the same content) will always produce a tone of the same pitch.
In general: The time period for one oscillation T or its frequency f (number of oscillations per time unit) does not depend on the amplitude.
This time period T is a property of the system, determined by the mass m of the oscillating object and the constant D of the restoring forces.
The simulation "10-oscillation-amplitude" demonstrates this statement.
10.5. Movement of a Pendulum
The movement of a pendulum is interesting under several different aspects.
- For a long time this movement was used to divide the continuous flow of time into equal intervals and thus to run clocks.
- The restoring forces, driving the pendulum back and forth, come close to the condition, which is set up for harmonic oscillations.
- Since a pendulum moves along a circle, centripetal forces are involved.
- A pendulum is driven by gravity, and therefore certain analogies to the motion of a freely falling body can be found.
All these aspects make the pendulum to an interesting object for studying these laws and phenomena in an integrated manner.
10.6. Mass of the Bob m and the Period for one Oscillation T
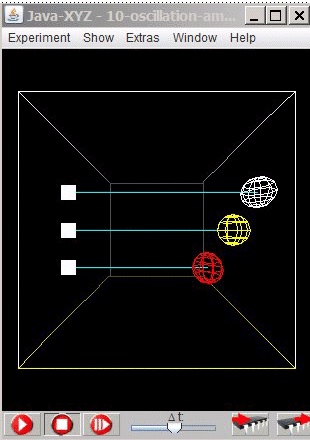
The two simulations "10-mass-Coulomb" and "10-mass-gravitation" are both simulating a movement of a pendulum. They differ in the kind of the acting force. In the first simulation the force is produced by an electrostatic field, oriented downwards (in -z-direction) acting on a charged bob. In the second simulation a gravitational field is acting on a neutral bob. Interesting and instructive is the difference which shows up, if the mass of the bob is changed. In the first simulation the mass has a strong influence on the period of the pendulum, in the second simulation the period is independent of the mass.
Fig 10.5.:Simulation "10-mass-Coulomb"
or "10-mass-gravitation"
The explication, why the period of a pendulum is independent of the mass of the bob is given by the influence of the gravitational mass. In case of a gravitational pendulum the gravitational mass compensates the influence of the inertial mass. In a pure electrostatic pendulum, however, the gravitational mass is missing and the allways existing inertial mass shows its influence.
The fact that the period of a pendulum is independent of the mass of its bob can easily be checked with rather simple experimental means.
10.7. Pendulum as ,Harmonic Oscillator"
Fig 10.6.: Simulation "10-pendulum-ho"
However, there is an interesting difference in respect to the influence of the mass of the swinging bodies.
If the mass of the swinging objects is changed, an important difference in behaviour shows up. Small changes of 20% or 40% are sufficient to see the difference.
Result: While the period T of the harmonic oscillator depends on the mass of the swinging objects, the period for the pendulum does not. Why?
The explanation can be found in the different type of force which drives the oscillating movement.
For the harmonic oscillator the elastic force of the spring remains the same when the inertial mass is changed.
For a pendulum the driving force is the gravitational attraction of the earth. This force is increased proportional to the inertial mass of the bob, because inertial and gravitational mass are strictly proportional. An enlarged inertial mass and an enlarged force neutralize themselves mutually and this is the reason why the period of the pendulum is independent of the mass of the bob.
10.8. Pendulum Period T and Length of Suspension
It was an important fact for building pendulum clocks, that the accuracy of the clock did not depend on the mass of the bob. Therefore the form and mass of this bob could have any seize and was free to be designed at will.
How could the accuracy of such a clock be changed, if it proceeded or followed?
The answer: By changing the length of the suspension.
Fig 10.7.: Simulation "10-pendulum-length"
|
|
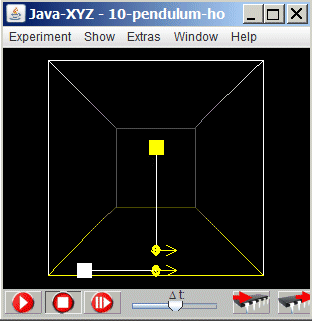
 When changing D and m, preferably in a systematic manner, the dependence of T on m and D (spring constant) can be derived and should, when ever possible, be checked by corresponding real experiments.
When changing D and m, preferably in a systematic manner, the dependence of T on m and D (spring constant) can be derived and should, when ever possible, be checked by corresponding real experiments.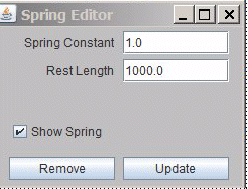 A spring can be set between two particles by
A spring can be set between two particles by 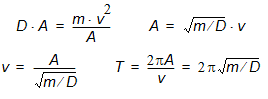
 As stated above, the time T needed for an oscillation is independent of the velocity with which an object starts from the equilibrium position.
As stated above, the time T needed for an oscillation is independent of the velocity with which an object starts from the equilibrium position.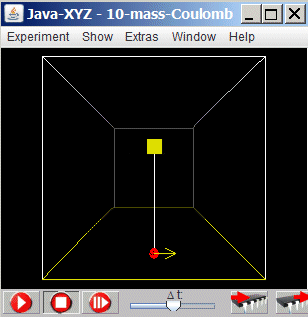
 The simulation "10-pendulum harmonic-oscillator"
The simulation "10-pendulum harmonic-oscillator"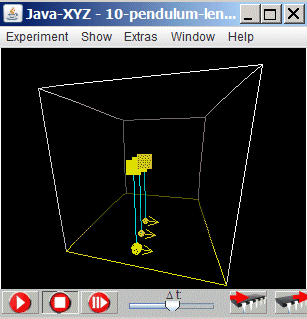 The prepared simulation "10-pendulum-length" shows three pendula which differ in length of the suspensions by 5% and 10% respectively.
The prepared simulation "10-pendulum-length" shows three pendula which differ in length of the suspensions by 5% and 10% respectively.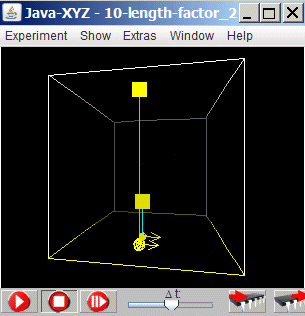 The ratio of the length of the 2 suspensions is
The ratio of the length of the 2 suspensions is